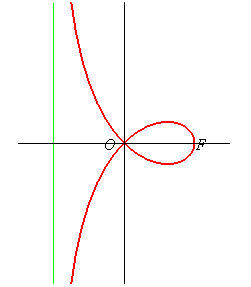
RIGHT STROPHOID (or NEWTON STROPHOID)

| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
RIGHT STROPHOID (or NEWTON STROPHOID)

| Curve studied by Roberval in 1645, Barrow (Newton's professor)
in 1670, and Jean Bernoulli; name given by Enrico
Montucci in 1837.
The name comes from the Greek Strophos "string, belt, braid". Isaac Newton (1642-1727): English mathematician, physicist and astronomer. Other names: pteroid (from pteron "wing"), cucumeïde (cucumion "broken pot") (connection?) and logocyclic. |
 |
Polar equation: Cartesian equation: Rational circular cubic with double point. Cartesian parametrization: Cartesian rational parametrization: If we consider the frame (F(0, a) (vertex of the loop), Length of the loop: Area of the loop: Area between the curve and the asymptote: |
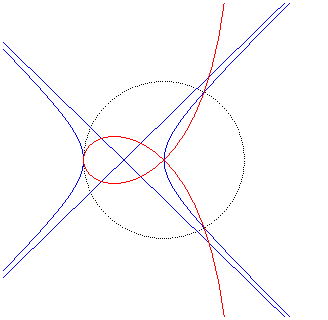
Given two points O and F, the right strophoid with vertex (or focus) F and centre O is the locus of the points M on a variable line (D) passing by F such that PM = PO where P is the intersection point between the line (D) and the line (D0) perpendicular at O to (OF) (called axis of the strophoid); in other words, it is the strophoidal curve of (D0 with respect to O and F (here, O is the origin of the frame and F is the point (a, 0)); it is a special case of the general strophoids. The point F is called focus because it is the singularity of this cubic.
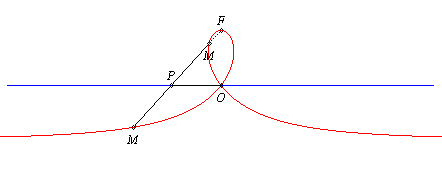 PM
= PO
PM
= PO| Therefore, the right strophoid is also the locus of the contact points of the tangents passing by F to the circles (in blue opposite) that are tangent at O to the axis (D0), or also the locus of the intersection points between the circles, orthogonal to the previous ones (in green opposite), tangent at O to (OF), and the lines joining F to their centres. | 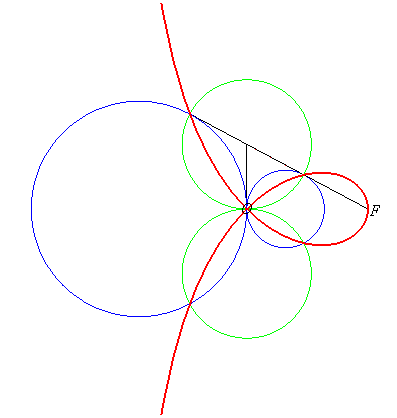 |
Here are two simple constructions:
| A variable line (D') passing by O cuts the line (D1) parallel to (D0) passing by F at Q; the strophoid is the locus of the point M where the circle centred on F passing by Q cuts (D'). | 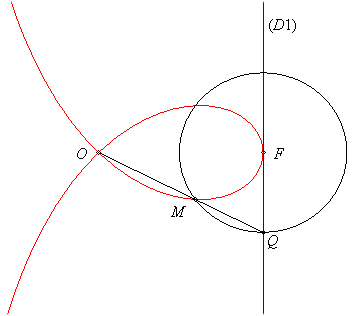 |
| Given a point |
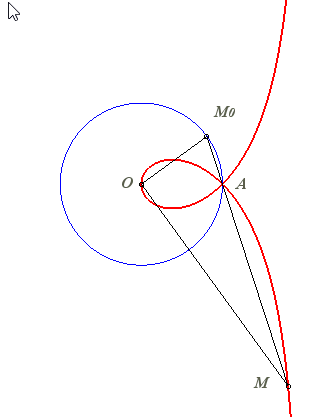 |
| The right strophoid is the locus of the orthocentre of
a triangle two vertices of which, A and B, are fixed while
the third describes a circles with radius [AB].
Cf. a similar construction of the bicorn. |
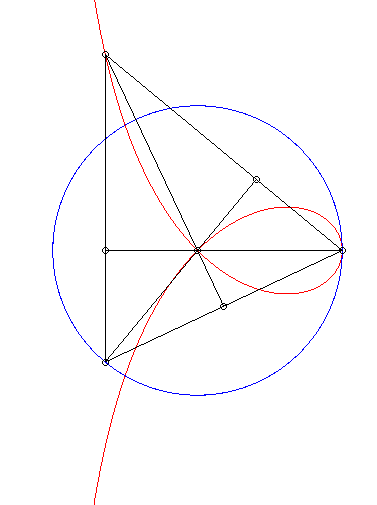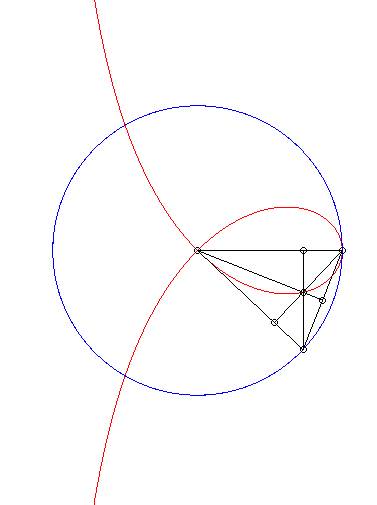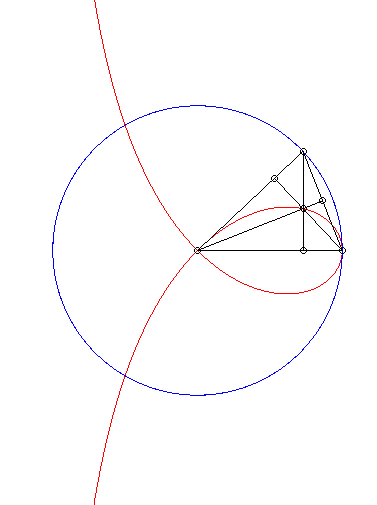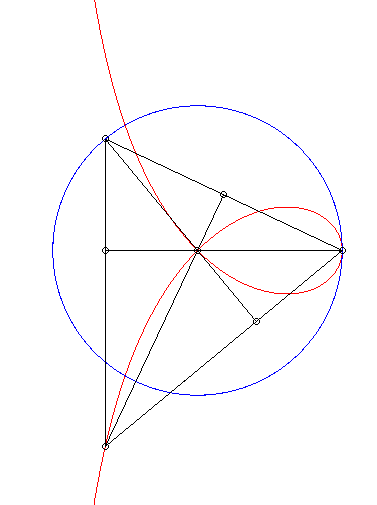 |
| If (C) is the circle with centre O passing by F and G is the point diametrically opposed to F, then the right strophoid is the locus of the points M such that, if P is one of the intersection points between the perpendicular to (OF) passing by M and (C), then the line (OM) and (GP) are parallel (construction of Rosillo). |
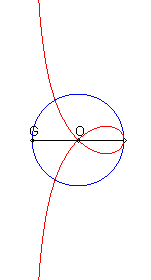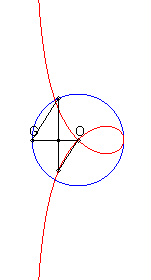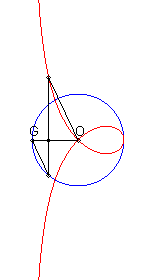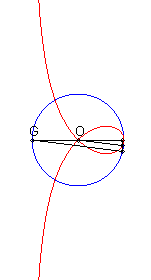 |
| The right strophoid also has the following elegant 3D
construction:
If (C) is the cylinder of revolution with axis (D0) and passing by F, then the right strophoid is the locus of the foci of the elliptic sections of the cylinder (C) by planes perpendicular to the plane of the strophoid and passing by F. This proves that the line (D0) is the median with pole F of the strophoid and itself. |
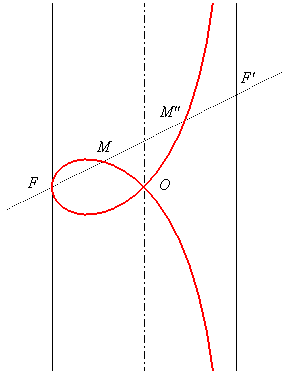
The foci of the ellipse with summits F and F' are M and M". |
| The polar equation |
 |
The complex equation above proves that for a line passing by F, the two intersection points with the strophoid are inverses of one another by the inversion with reference circle the circle (C) with centre F passing by O:
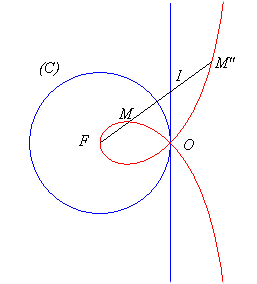
Like all rational circular cubics, the right strophoid can be defined as:
- the cissoid
with respect to O of a circle with radius a passing by O
and a line parallel to the tangent at O and located at distance
a
from the circle (the asymptote of the strophoid); here the circle (C)
passing by O with centre F:
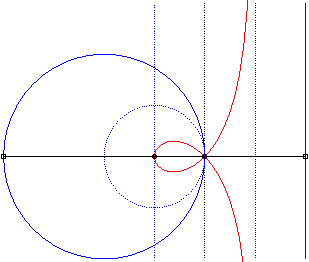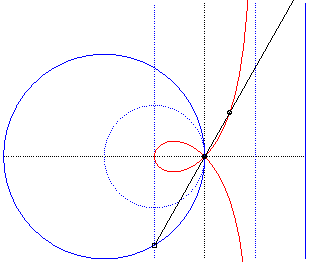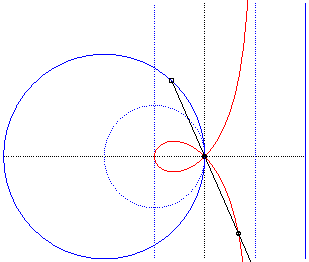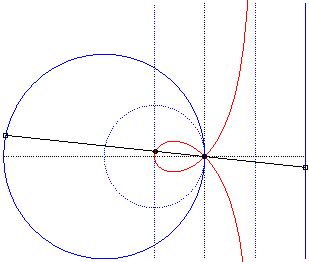 |
The strophoid is the cissoid of the dotted circle and external line, hence also the median of the full circle and line, homothetic images of the previous ones. |
l
 |
Therefore, the right strophoid is the envelope of the circles with diameters joining O to a point on the parabola. In other words it is a cyclic curve with deferent (or initial curve) a parabola, a radius of inversion equal to zero and a pole at the foot of the directrix of the parabola. |
- the inverse
of a rectangular hyperbola with
respect to one of its vertices (here the rectangular hyperbola with vertices
O
and F (equation ),
with respect to O).
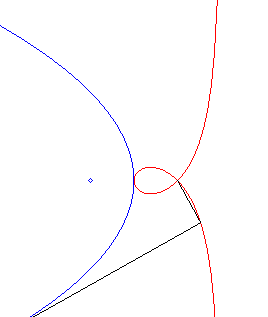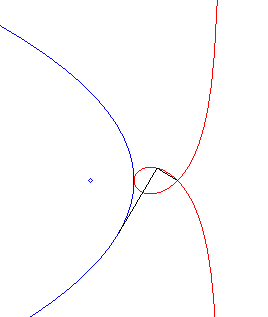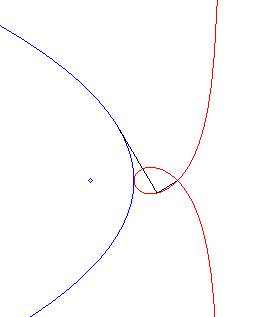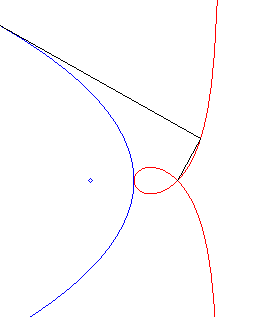
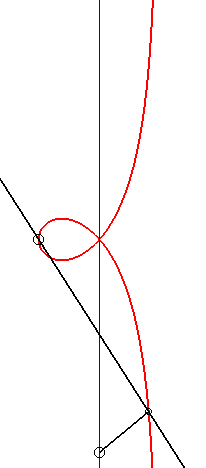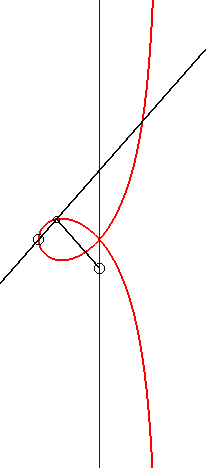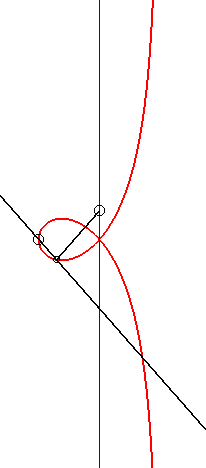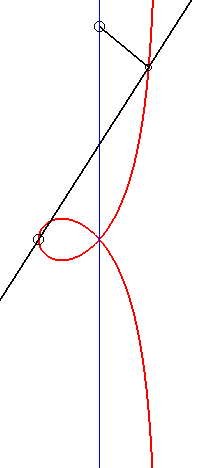
| - by the Newton set-square method: |
|
 |
 |
The right strophoid is also:
- a special case of nodal
curve (cf. the second polar equation).
- a special case of cubical
hyperbola (cf. the Cartesian equation)
| - a special case of Maclaurin
sectrix, hence the construction with 2 lines in uniform rotation, one
of which going twice as fast as the other.
Application: the locus of the centre of the circle inscribed in the triangle ABC when C describes the circle with centre A and radius AB is a right strophoid. |
 |
Finally, it has a mechanical definition as a curve of the tightrope walker:
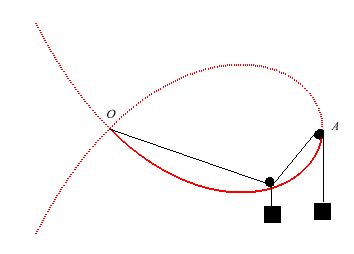 |
A tightrope walker walks on a rope attached to a fixed point O, passing by a pulley A located at the same height than the attached end, and tightened by a counterweight attached to the other end, the weight of the walker being equal to that of the counterweight. |

| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2024