Seam line of a tennis ball

SEAM LINE OF A TENNIS BALL

View above made with povray by Alain Esculier.
Website in German.

What is the curve described by the seam line of a tennis ball?

It seems natural to require certain conditions:

1) it must be traced on a sphere (for example with center O)

2) it must be invariant under the action of a half-turn

3) it must divide the sphere into two pieces that are swapped by the aforementioned half-turn.

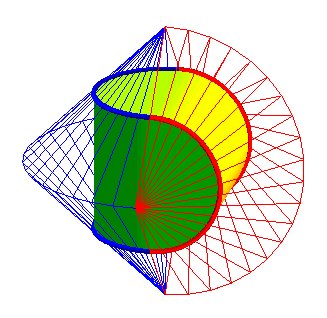
Under these conditions, a possibility consists in the reunion of 4 semicircles presented opposite. This curve is the contact curve of the inscribed sphere of a sphericon, a surface composed of 4 portions of cones of revolution.

But the curvature of this curve is not continuous, and one may wonder if the following condition can be added:

4) it must be rational

The answer is yes: the view 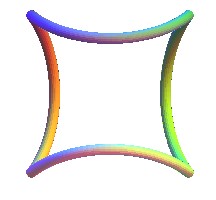gives the idea to consider the hypotrochoid with symmetry of order 4 as the projection on xOy, and an adequate sinusoidal function for z. We get the curve:

Cartesian parametrization: .
Curve traced on a sphere (with center O and radius d = a+b) iff .
Rational curve of degree 6.
The 3 axes are axes of symmetry under a half-turn, but only Ox and Oy swap the two portions of the sphere defined by the curve.

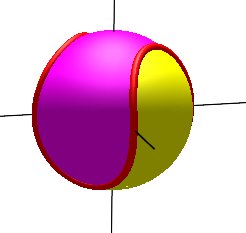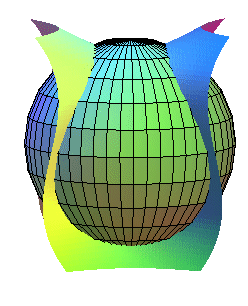
Opposite the distortion of the curve, which is a special case of the satellite curve, for a constant value of d and a ranging from d/2 to d.
The case b = a = d/2 (double point at the north pole) gives a clelia, and its view from above is a quatrefoil curve.
A little after d/2, the curve goes through a phase where it looks like the curve of a basketball (disregarding the two great circles traced on the actual ball).

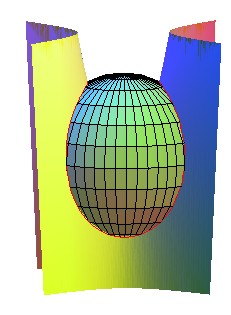
The value of b for which the curve has a vertical tangent at the intersection point with the equator is b = a / 3 (the projection is an astroid). It corresponds more or less to the actual curve on a real tennis ball.
This curve, parametrized by , is the intersection of two cylinders with perpendicular axes:.

Various views of the curve for b slightly smaller than a. This curve is the intersection between the surface and a cubic surface: it is an algebraic curve of degree 2x3 = 6.

But can we find a curve satisfying all the conditions from 1) to 4)?

On www.gebr-grimm.de/cucke/seite2.htm, the name "Tennisballkurve" is given to the intersection between the sphere and the hyperbolic paraboloid . This curve has the advantage of being of degree 4 (it is a spherical biquadratic), but it does not satisfy property 4): it is not rational.
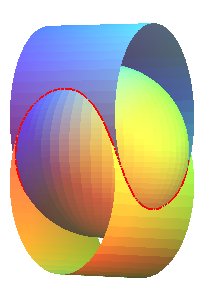It is also the intersection between the two elliptical cylinders and where (see this website where it is called Bishop curve).
This curve is therefore a dilatation of a bicylindrical curve.

Another possibility is the spherical sinusoid with two arches, intersection between a half sinusoidal cone and a sphere.
It is one half of an algebraic curve of degree 8.
The comparison shows that this curve (in red) is less harmonious than the previous curve (in blue).

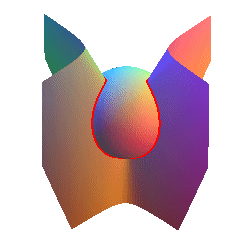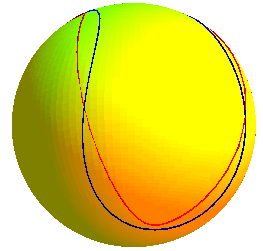Another possibility is the intersection between the Plücker conoid and a sphere with diameter greater than the "height" of the conoid; it is an algebraic curve of degree 6, not rational.

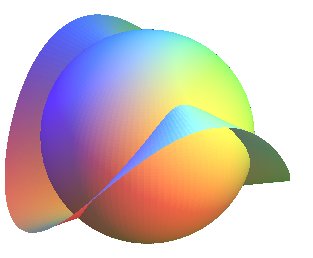The seam line divides the surface of the ball into two equal portions, but the cone, with vertex the center of the ball, supported by the seam line also divides the inside of the ball into two equal portions!
It is the idea used by the sculptor Patrick Collandre to develop in three dimensions the classical symbol of yin and yang.
His separation curve is composed of 4 circular arcs.
He called his sculpture: tao sphere.

But let us come back to the seam line curve seen as the contact curve between the sphericon and its inscribed sphere. The convex hull of the seam line is then composed of two portions of cylinders and 4 half-disks: it is the reciprocal polar of the sphericon with respect to this sphere. Tadeusz Dorozinski called it: sphericylinder.

Remark: the tennis ball theorem states that any smooth curve different from a circle dividing the surface of a sphere into two isometric parts has at least 4 inflection points.

See also this article on the design of a base-ball cover.

Artistic view of a curve that strongly resembles the view from above of the seam line of a tennis ball.

Sculpture by Vieweger located at the entrance of a tennis club in Munich.

next curve previous curve 2D curves 3D curves surfaces fractals polyhedra


Various views of the curve for b slightly smaller than a.	This curve is the intersection between the surface and a cubic surface: it is an algebraic curve of degree 2x3 = 6.

On www.gebr-grimm.de/cucke/seite2.htm, the name "Tennisballkurve" is given to the intersection between the sphere and the hyperbolic paraboloid . This curve has the advantage of being of degree 4 (it is a spherical biquadratic), but it does not satisfy property 4): it is not rational. It is also the intersection between the two elliptical cylinders and where (see this website where it is called Bishop curve). This curve is therefore a dilatation of a bicylindrical curve.
Another possibility is the spherical sinusoid with two arches, intersection between a half sinusoidal cone and a sphere. It is one half of an algebraic curve of degree 8. The comparison shows that this curve (in red) is less harmonious than the previous curve (in blue).
Another possibility is the intersection between the Plücker conoid and a sphere with diameter greater than the "height" of the conoid; it is an algebraic curve of degree 6, not rational.