JERABEK CURVE

| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
JERABEK CURVE

| Curve studied by Jerabek and Neuberg in 1885.
Loria p. 239. |
 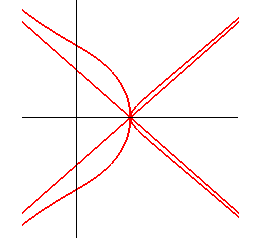 |
Polar equation: For 0 < k = tha <1: For k = cotha > 1: Cartesian equation: Polar equation in the frame centred on (a,0):
|
| The Jerabek curve Therefore, it is the base of the movement of a plane over a fixed plane a fixed point of which is P and a fixed line of which is (PA), this movement is called circular conchoidal movement. |
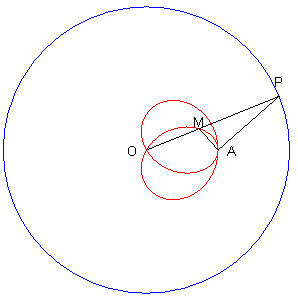 |
When A is inside the circle (k > 1), the curve is closed, included in the circle, and has a crunodal double point and a tacnodal double point.
When A is outside the circle, there is a tacnodal double point and two asymptotes.
Besides, these two cases are inverses of one another:
more precisely, the curves
and
are
inverses of one another with respect to the circle (C).
Writing the polar equation
where e = 1/k, proves that the Jerabek curve is the conchoid
of a centred conic with pole a focus of this conic, and modulus the semi-major
axis (see conchoid of
a conic).
It is also the pedal
of the evolute of a centred conic with respect to one of its foci.
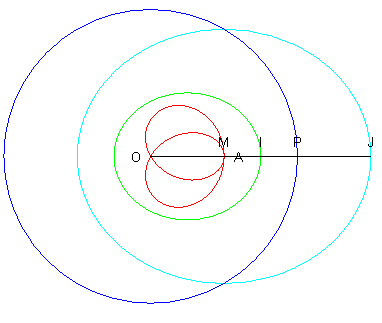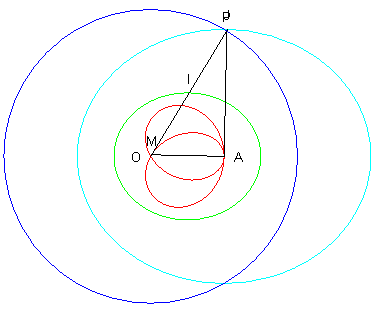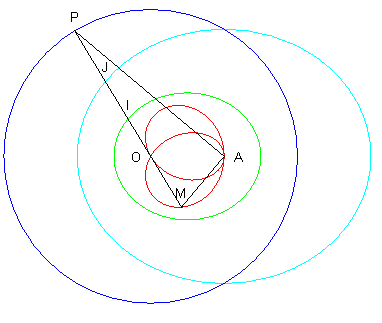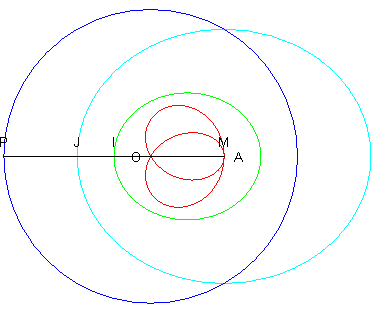
| When A is inside the circle, if I is the
middle of [MP] , IM = IP = IA, so OI
+ IA = OP = constant: the point I describes an ellipse and
the Jerabek curve can be obtained by decreasing the radius vector of
the green ellipse starting at the focus O by a length equal to the radius
vector that reaches the other focus A.
Let J be the image of I by the homothety with centre O and ratio 2; J describes the blue ellipse and JM = OP = constant: the Jerabek curve is the conchoid of the blue ellipse with respect to the focus O, the radius vector being decreased by the semi-major axis of this ellipse. M is the symmetrical image of A with respect to the normal to the green ellipse passing by I: the Jerabek curve is therefore the orthotomic with pole A of the involute of the evolute of the green ellipse. See strophoidal
curves, for a strophoidal construction of the Jerabek curve using this
property.
|
 |
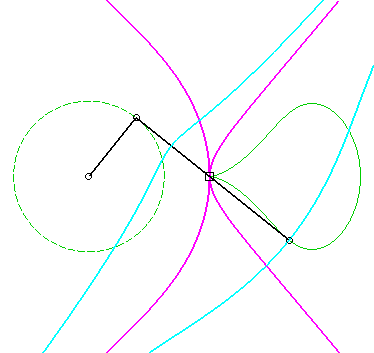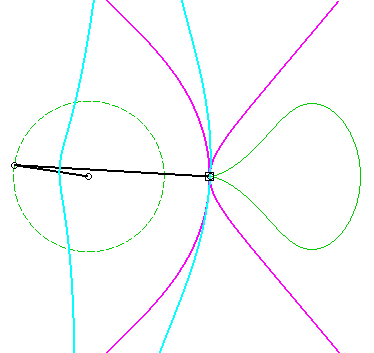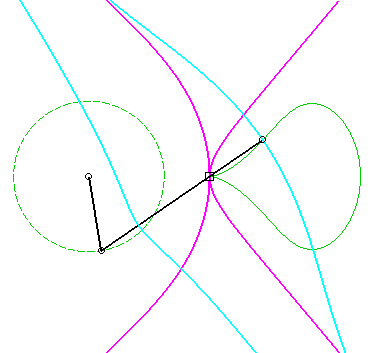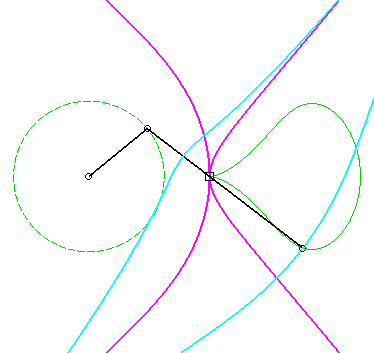
The Jerabek curve is the base of the circular conchoidal movement.
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2017