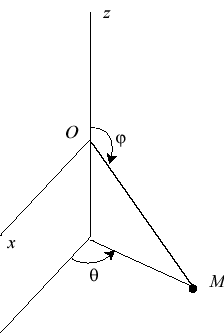
Differential equation of the motion (derived from Newton's second law): . Translation in spherical coordinates:  where q = longitude, j = colatitude and . First integral: . Spherical differential equation of the curve:  (with ), i.e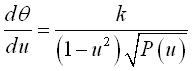., with :  where , which leads to an elliptic integral; note the tiny difference with the spherical catenary, for which .

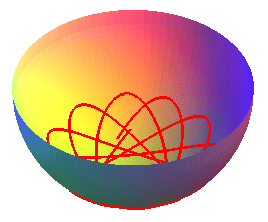
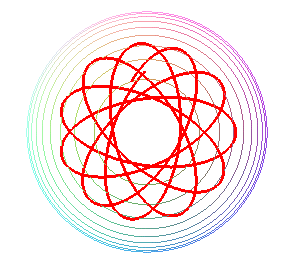
The curve of the spherical pendulum is the curve described by the end of a simple massive pendulum attached to a fixed point, that can move in three dimensions, and placed in a uniform gravitational field (here ).

This curve is traced on a sphere, and is none other than a flow line of this sphere: it can be physically obtained by making a ball roll inside a sphere.

Like the case of the spherical catenaries, we get curves composed of a sequence of undulations joining alternatively two parallels (obtained for the values at which the polynomial P above cancels), and images of one another by rotations around Oz. The curve is either closed, or dense in the zone between the two parallels.

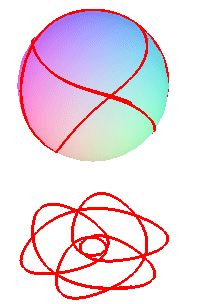These acrobat bikers of the Shanghai circus describe such curves.
 

These curves can be generalized by considering the Coriolis force, which give the following differential equation of the motion: .

When the pendulum is dropped without an initial speed, we get the curve of the Foucault pendulum, which, for small oscillations, can be approached by a hypocycloid.
Without the Coriolis force, the curve would amount to an arc of a circle.
 
 

When the vector W is not vertical, the curve no longer has rotation symmetry around Oz.

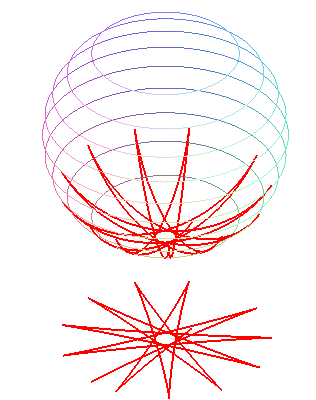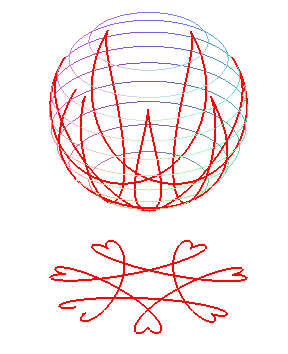Here is a view of the curve described by a pendulum in forced uniform rotation around Oz.

See the spinning top festoons, which are another generalization of the curves of the spherical pendulum.
 

next curve

previous curve

2D curves

3D curves

surfaces

fractals

polyhedra

© Robert FERRÉOL  2018